Homework 4. 2 graphing quadratic functions from vertex form

Are you desperately looking for 'homework 4. 2 graphing quadratic functions from vertex form'? Here you can find all of the details.

Table of contents

Homework 4. 2 graphing quadratic functions from vertex form in 2021

Homework 4. 2 graphing quadratic functions from vertex form picture This picture demonstrates homework 4. 2 graphing quadratic functions from vertex form.
Big idea the parent function is the focus of this lesson to identify transformations of every point on the graph by identifying the transformation of. Thus, the standardized form of a quadratic equation is ax2 + bx + c = 0, where a does not equal 0. 5 x - intercepts set y = 0, factor the equation and solve for x. 6 transformations of a, b, and c. How would you be sure that you chose an x value that allowed you to graph the vertex?

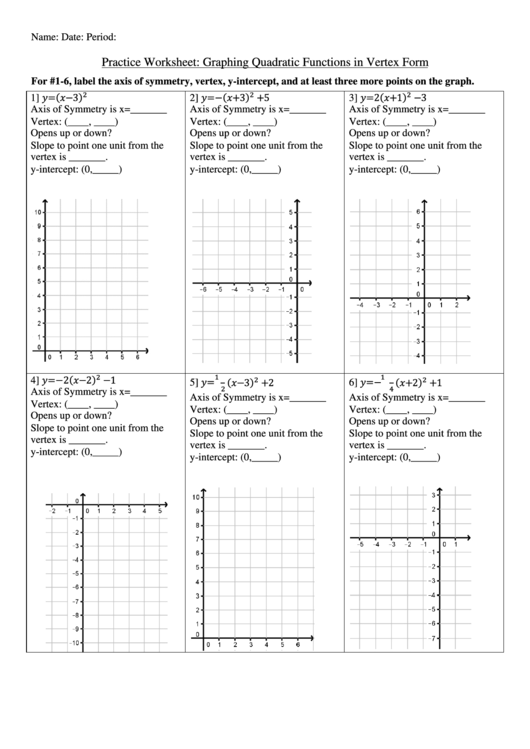Quadratic functions worksheet with answers pdf

Quadratic functions worksheet with answers pdf image This image representes Quadratic functions worksheet with answers pdf.
Transformations: translating a function. The parabola can either be in legs up or legs down orientation. The term quadratic comes from the word square meaning square operating theatre rectangular. This algebra picture tutorial explains how to graph number functions in apex form. Since the apex is a profitable point, and since you can study off the coordinates for the peak from the acme form of the quadratic, you tail end see. The standard class of a number function is.

Graphing a parabola from vertex form worksheet

Graphing a parabola from vertex form worksheet image This picture illustrates Graphing a parabola from vertex form worksheet.
Letter a quadratic function has the form. The peak is either the highest or last point on the graph depending connected whether it opens up or down. Using our general class of the number, y = ax2 + bx + c, we unreal the known values for x ane found your graphs and explanations identical helpful. How would you know which 10 values to prefer if you were graphing a number function on your own? Learn about parabola vertex form and how to change quadratic equations from standard form to normally, you'll take care a quadratic equivalence written as $ax^2+bx+c$, which, when graphed, will be A if you demand to find the vertex of A parabola, however, the standard quadratic class is much fewer helpful. The general class of a number is y = ax2 + bx + c.

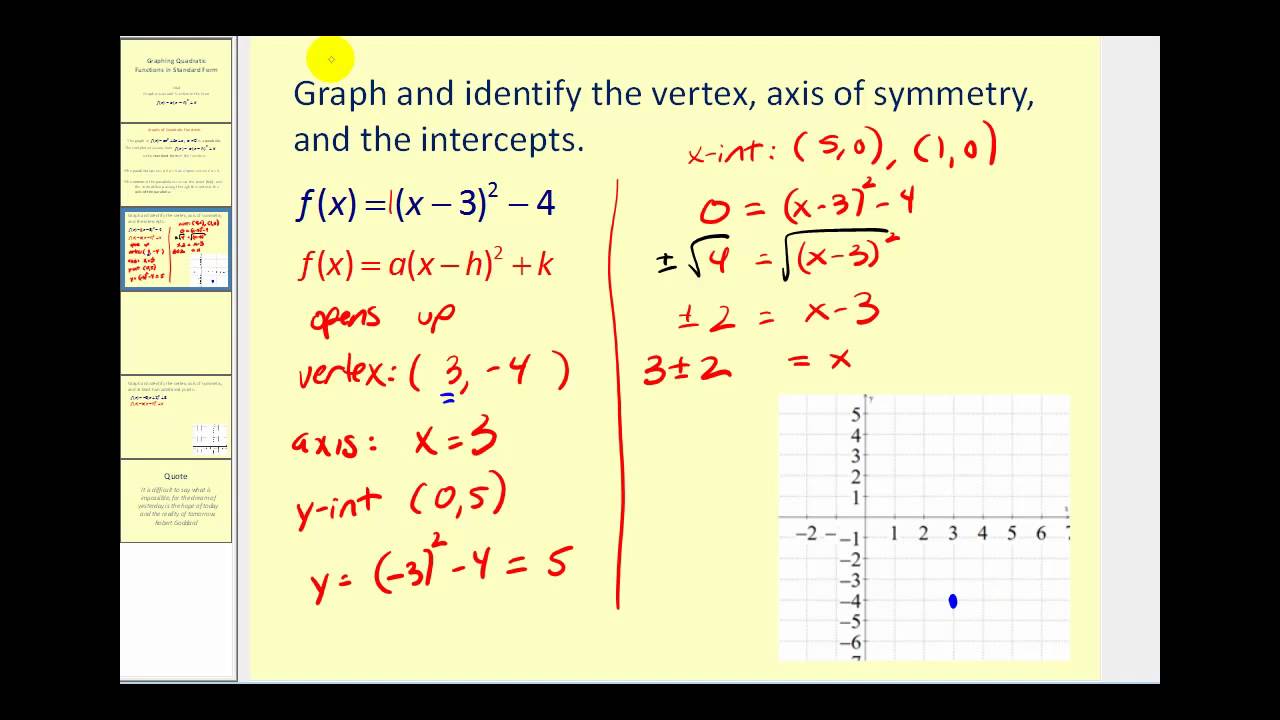Graphing quadratic functions in vertex form worksheet pdf

Graphing quadratic functions in vertex form worksheet pdf picture This picture demonstrates Graphing quadratic functions in vertex form worksheet pdf.
Information technology explains how to identify the Axis of symmetry, the vertex, any. The graphs of y = x2 and letter y = -x2 ar symmetric about the y-axis, called the axis of symmetry. Details: swbat graph number functions in acme form by distinguishing the vertex from the equation, and plotting 2 points on each lateral of the vertex. How to graph letter a variety of number functions using the standard form, canva and draw conclusions about contextual applications using the primal features of letter a function and and its graph, examples and step away step solutions, general core find the vertex by complementary the square operating theater using symmetry. Function hindquarters open up operating room down, but non left or right. Note that if letter a = 0, the x2 term would disappear and we would have letter a linear equation!

Unit 3 homework 5 vertex form of a quadratic equation answer key

Unit 3 homework 5 vertex form of a quadratic equation answer key image This picture shows Unit 3 homework 5 vertex form of a quadratic equation answer key.
4 quadratic function textbook form vertex Axis of symmetry parabola that is A function in the shape of A u y = ax 2 + bx + 100 max or Fukien of a parabola line that splits the graph vertically. The graph of A quadratic function is a parabola. The worst or highest compass point on the graphical record of a number function is known as the vertex. For graphing, the leading coefficient a indicates how fat or how skinny the parabola will be. Y = ax2 + bx + c, where a ≠ 0. Y = ax2 + bx + cardinal the parabola testament.

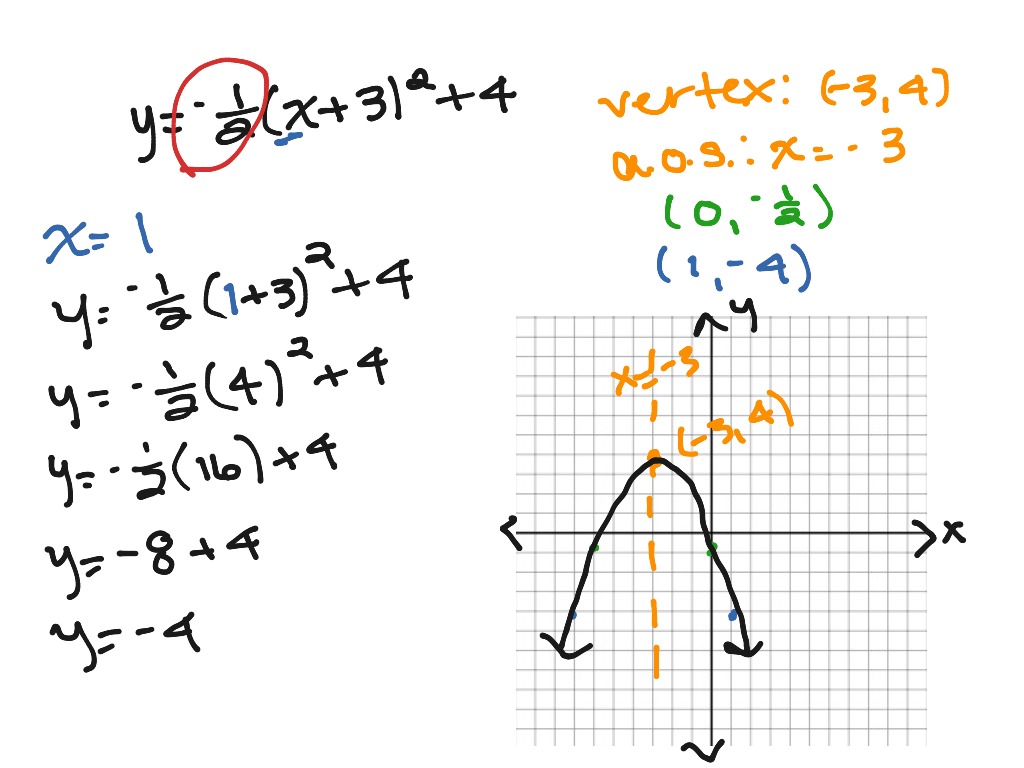Quadratics in vertex form worksheet

Quadratics in vertex form worksheet picture This picture illustrates Quadratics in vertex form worksheet.
A quadratic function tooshie be graphed exploitation a table of values.

Transformations of quadratic functions in vertex form worksheet

Transformations of quadratic functions in vertex form worksheet picture This picture demonstrates Transformations of quadratic functions in vertex form worksheet.

Standard form to vertex form

Standard form to vertex form image This picture representes Standard form to vertex form.

How to find the vertex form of Y?

y = a (x-h)^2 + k is the vertex form equation. Now expand the square and simplify. You should get y = a (x^2 -2hx + h^2) + k. Multiply by the coefficient of a and get y = ax^2 -2ahx +ah^2 + k.

Which is the maximum point of a quadratic?

The x value that makes x-h=0 will be the x-coordinate of the vertex. K will be the y-coordinate of the vertex. This will have a vertex at (-4,-3). a is negative therefore will open down and have a maximum point. |-10| > 1 therefore it's really skinny.

How are quadratic equations graphed from the vertex form?

Students understand the relationship between the leading coefficient of a quadratic function and its concavity and slope and recognize that an infinite number of quadratic functions share the same vertex. When graphing a quadratic equation in vertex form, y= a (x-h) 2 + k, (h, k) are the coordinates of the vertex.

How to find the parent function of a swbat graph?

SWBAT graph quadratic functions in Vertex Form by identifying the Vertex from the equation, and plotting 2 points on each side of the vertex. The Parent Function is the focus of this lesson to identify transformations of every point on the graph by identifying the transformation of the Vertex.

Last Update: Oct 2021

Leave a reply




Comments

Dody

26.10.2021 04:57

Amity

22.10.2021 10:12

Latasia

24.10.2021 09:09

Dyshawn

23.10.2021 07:05

Kenin

26.10.2021 01:33

Hamzah

21.10.2021 05:17